Introduction to Nuclear Criticality Safety
REVIEW TOPICS PART II
Part II continues our review of foundational knowledge.
NEUTRONS AND THEIR INTERACTIONS
Nuclear reactions are the only sources of neutrons since neutrons do not exist in nature. The most common neutron sources, if we don't count fission as a neutron source (just for the time being), depend on ( 
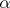,n) and ( 
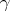,n) reactions with light element. Examples of these reactions are (Bennet & Thomson 1988, p. 23):
9Be + 4He 
12C + 1n
2H + 
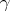
1H + 1n
The average lifetime of a neutron is approximately 12 minutes. However, in a nuclear reactor the average lifetime of a neutron is only a small fraction of a second. Since a neutron is neutral (i.e., uncharged), it does not undergo a force of repulsion when it approaches a nucleus. This makes it possible for neutrons of any energy to interact with nuclei, although low energy neutrons tend to interact more readily than high energy neutrons. We will discuss this some more later. Now, the three most important neutron-nucleus reactions are:
- Radiative Capture,
- Scattering (elastic or inelastic), and
- Fission.
These processes are commonly divided into two groups (based in the principal mechanisms of interaction between the neutrons and nuclei). The groups are:
- elastic scattering and
- compound nucleus formation.
In elastic scattering the incident neutron scatters elastically off of the nucleus without actually penetrating the nuclear surface. Compound nucleus formation includes the processes for the neutron being captured in the nucleus, being scattered, or causing the fission of the nucleus. Let's now describe these processes.
Elastic Scattering
Scattering does not change our neutron population, but is does alter the velocity (energy and direction) of the neutron. Elastic scattering alters the kinetic energy of the nucleus (through transfer of kinetic energy from the neutron to the nucleus), but not its internal energy level (nucleus remains unchanged in its ground state). The incident neutron is bounced off the nucleus in a similar fashion to the collision of two billiard balls of unequal mass. Elastic scattering (n,n) can be described as:
It often happens that the incident neutron has a higher kinetic energy than the nucleus, which as a result of the interaction causes the neutron to emerge with reduced kinetic energy. This can mathematically be described as following. (Note: both energy and momentum is conserved in the elastic scattering interaction)
A neutron of mass m is moving with velocity v1 towards a nucleus at rest (i.e., stationary) of mass M. Consider this situation as part of the laboratory system (L system), which is illustrated below.
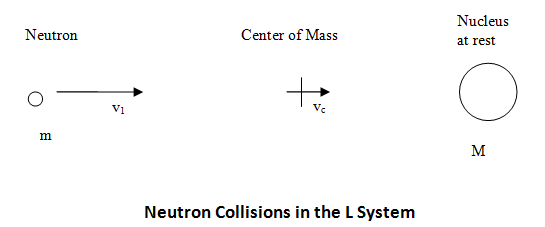
In the L system the center-of-mass is moving towards the stationary nucleus with a velocity given by ( Bennet & Thomson 1988, equation 2.1):
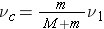or 
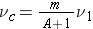
(since atomic masses are close to the same as mass numbers)
Now, let's adopt a system of coordinates where the center-of-mass of the neutron-nucleus pair is at rest and refer to this as the center-of mass system (CM system). In order to cause the center-of-mass to become stationary (or at rest), we need to impose a velocity equal in magnitude and opposite in direction to v c on the whole system, which becomes transformed to that shown below.
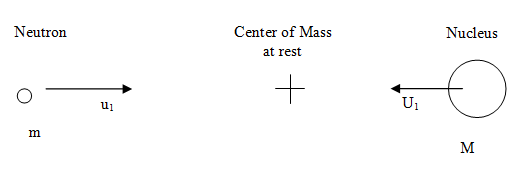
Neutron Collisions in the CM System
The neutron and nucleus are now depicted in the CM system of coordinates and their velocities are respectively (Bennet & Thomson 1988, equations 2.2 & 2.3):
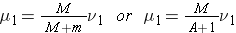
and
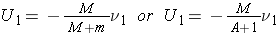
Note that mu1 + MU1 = 0, which means that the momentum of the neutron-nucleus pair is zero in the CM system (and it will remain zero after any reaction for which the law of conservation of momentum is valid).
The total kinetic energy of the two particles in the CM system before any interaction is expressed as (Bennet & Thomson 1988, equation 2.5) :
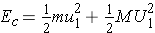
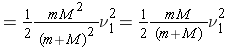
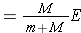
where E is the kinetic energy of the neutron in the L system.
After an elastic scattering collision the momentum of the neutron-nucleus pair in the CM system is zero and their kinetic energy is unchanged. In order for the momentum to remain zero after an elastic scattering collision, the neutron and nucleus must move away from the location of the collision in opposite directions with velocities u2 and U2 respectively (i.e., mu2 + MU2 must equal zero).
To conserve kinetic energy, the kinetic energy before the collision must be equal to the kinetic energy after the collision. This can be expressed as ( Bennet & Thomson 1988, equation 2.7):
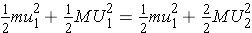
It can then be concluded that U1 = U2 and also that u1 = u2 , which indicates that the speed of neutron and nucleus in the CM system are unchanged, regardless of scattering angle, in an elastic scattering collision.
Now, to find the effect of the collision on the neutron speed in the L system, which is really what we are after, we will revert to the L system by imposing on the CM system a velocity vc (same vc as used before to describe the velocity of the center-of-mass in the L system). The speed of the neutron after an elastic scattering collision in the L system can be found by adding the velocity vc to the velocity u2 and using the cosine rule:
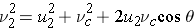
where q is the angle of scattering in the CM system, as illustrated below.
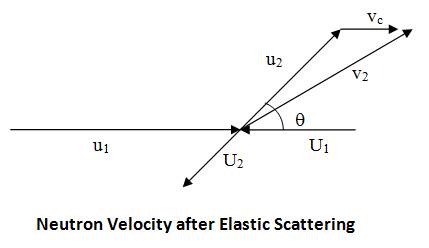
Using our previously defined expressions for v c , u 1 and recalling that u 1 = u2, the speed of the neutron after an elastic scattering collision can now be expression as ( Bennet & Thomson 1988, equation 2.10) :
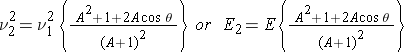
These expressions tell us that the neutron speed or energy after elastic scattering is a function of:
- Energy before collision
- Mass number of the scattering nucleus, and
- Angle of scattering in the CM system.
If we have a head-on collision (i.e., 
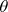=180 ° ) and as a result the neutron bounces back along its original path, then cos 
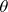= -1 and consequently
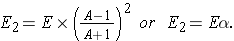
a is called the collision parameter and is defined as (Id., equation 3.30)
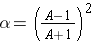
The fractional loss of energy is given by (Id., equation 3.32):
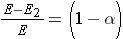
It can be seen from the expressions above that as the mass number decreases, the loss of energy as a result an elastic scattering collision increases. A neutron can lose all its energy to the hydrogen nucleus in a single head-on collision (A = 1, 
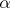= 0).
