Introduction to Nuclear Criticality Safety

REVIEW TOPICS PART IV

Continuing . . .

MEAN FREE PATHS

The average distance that a neutron travels without interacting is known as the mean free path.   We can define a probability density ( ≈ distribution) function for first collision in our beam problem:

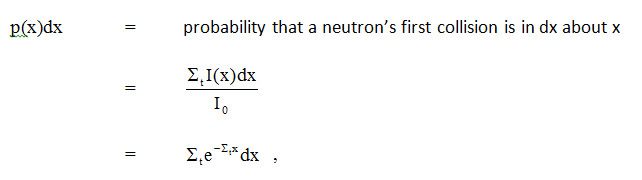
ProbabilityFirstCollision.png

which means the probability density function (pdf) for first collision is p(x) = 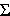te-t x. We can use this pdf to obtain averages.   For example:

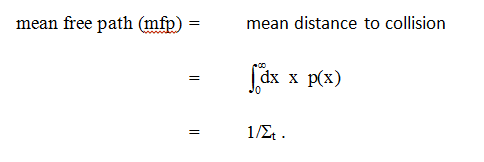
MeanFreePath.png

The mean free path is the reciprocal of the macroscopic cross section. It follows from their definitions that macroscopic cross sections are additive in the same way that microscopic cross sections are:

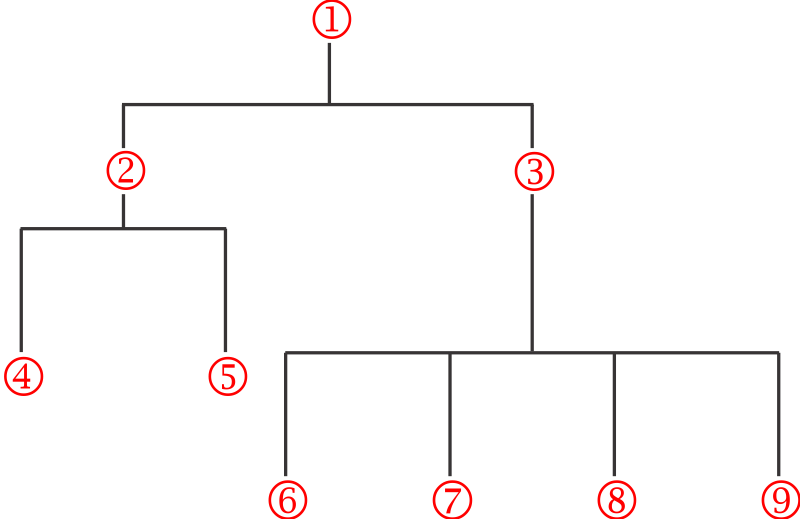
Match the numbers within the hierarchy with the proper terms: Σt Σs Σa Σin Σe Σs Σn,2n Σn,α Σ

NeutronHierarchy.png

 

Value: 9

Match the items.

The task is to match the lettered items with the correct numbered items. Appearing below is a list of lettered items. Following that is a list of numbered items. Each numbered item is followed by a drop-down. Select the letter in the drop down that best matches the numbered item with the lettered alternatives.

a. Σn,α

b. Σs

c. Σ

d. Σin

e. Σe

f. Σn,2n

g. Σf

h. Σt

i. Σa

 

In addition, given:

a mixture of nuclides:                     YA, YB, ....,

with number densities:                   NA, NB, ....,

and microscopic cross sections:     σx A, σx B, .....,

then for any type, x, of interaction, the macroscopic cross section for the mixture is:

Interaction.png


return to top | next page