Introduction to Nuclear Criticality Safety
REVIEW TOPICS PART III
Continuing . . .
MICROSCOPIC CROSS SECTIONS
Microscopic cross sections describe the likelihood that a neutron will interact with a given nucleus. Consider a beam of neutrons, all with the same velocity, normally (perpendicularly) incident on a very thin plate of some material as illustrated in the figure below. Assume that the target is so thin that all nuclei "see' the same beam intensity. (This is a limiting case - we can only approach this "thin limit" with very thin targets.)
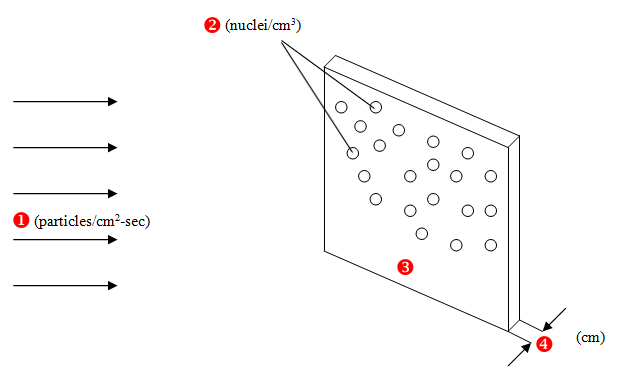
The rate at which neutrons collide with nuclei must be proportional to IA (the rate at which neutron strike) and to N D x (the areal density of target nuclei). Putting this statement into mathematical language, and using the symbol s to represent the constant of proportionality, we get the following:
Rate = δΙΑΝ∆x, or
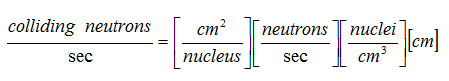
We have determined that s must have units of area (or to be exact, area per nucleus). The symbol s is referred to as a "microscopic cross section" and is tabulated in units of barns (1 barn = 10-24 cm2). It might be useful to think of σ as the effective area that a nucleus presents to a neutron.
Earlier we described s as a "constant" of proportionality. However, if we changed anything about our simple experiment, we might expect our "constant" to change. That is, we might expect to have a different σ for different neutron speeds and for different target nuclei. In fact, microscopic cross sections depend on:
- the [_____] speed between the neutron and the nucleus, and
- target nuclide.
In the preceding discussion we were looking at the total rate at which neutrons interact with nuclei, without distinguishing between kinds of interactions (elastic scattering, fission, inelastic scattering, capture, etc.). The σ that we used above is called the microscopic total cross section, and is denoted σt. It can be thought of as the sum of microscopic cross sections for various specific interactions:
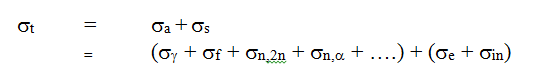
where . . .
We can also assign separate cross sections to characterize elastic scattering σe in which the target nucleus remains in its ground state, and inelastic scattering σin in which the target nucleus is left in an excited state. The scattering cross section is therefore the sum of the elastic and inelastic scattering cross section (Id., p. 55; Duderstadt & Hamilton 1976, p. 18):
σs = σe + σin
Note that cross sections are related to probabilities of various types of reactions.
Based on the previous discussion, the neutron cross section hierarchy is illustrated below. Match the circled numbers (1-9) with the correct labels.
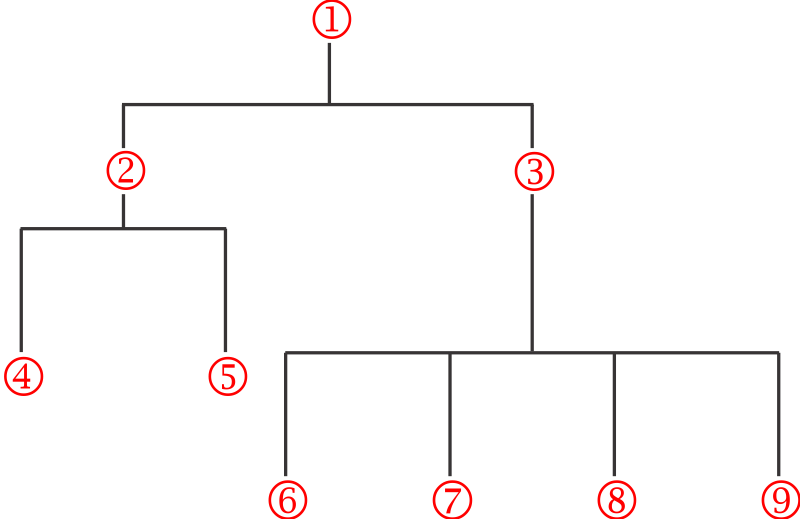
NOTE: Some answers below are interchangeable. However, machine scoring cannot account for this. If your answers appear incorrect, you can reverse your answer or simply ignore the score. (You get to report your better score when you submit your review credit.)


